In the run up to the National tests for eleven year olds called SATs this May, I was practising with some of my pupils what some of the question would look like.
The girl looked at the question and said: “there’s an easy way of doing this.”
The question said 56 ÷ 4 =
It is one of those rare questions in a Key Stage 2 SATs paper that requires a simple answer to a mathematical expression. The girl I’m sure had seen that question every year for the last five years. Yet she was still hesitant – she had no instant response to the question. She had to think of the ‘easy way‘. And unfortunately she went on to choose the wrong easy way.
“My teacher told me you just drop the ‘6’ off the end, add one on to the 5 and that’s the answer.” Unfortunately the girl was remembering the ‘easy way’ for dividing by 9. And she was remembering the answer to the expression 54 ÷ 9 (which of course is 6).
This one of the reasons I dislike teaching children easy ways of doing things. In my experience most children who are taught easy ways have learned the underlying principles behind them. They then can only remember a small number of many easy ways and eventually they forget which way is which and when to use it. The next step is to decide that they can’t do mathematics anymore and they switch off from the subject altogether.
To quote a biblical metaphor, it’s a bit like building your house on the sand. It only takes a single storm of confusion to reveal that there were no foundations and everything is washed away.
Putting it another way, it’s like badly applying Bloom’s taxonomy to teaching. 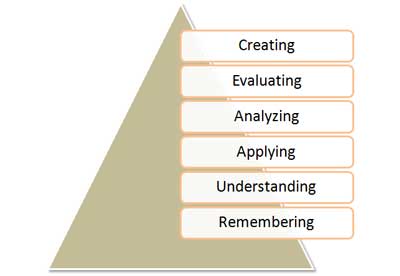 It seems we’re very keen in the teaching world at the moment to find ways of teaching those higher skills of evaluating and creating. But we miss the vital step between remembering things and applying them – that of understanding them.
It seems we’re very keen in the teaching world at the moment to find ways of teaching those higher skills of evaluating and creating. But we miss the vital step between remembering things and applying them – that of understanding them.
We teachers often talk about that ‘wow’ moment in lessons – that realisation by the students that they are really ‘getting it’. This most often happens in 1:1 interactions but can also happen with larger groups. When I look at the Bloom’s Taxonomy chart I would say that that ‘wow’ moment comes in the ‘understanding’ phase. It’s not when we’re sure children can remember things by heart, or when we see them diligently applying their knowledge, nor even we see the outcome of a great piece of creativity. It’s when children comprehend, when you can look into their eyes and know they have understand – when they get it.
So, back to the girl with the maths problem.
Striving for that moment of understanding, I asked, “are you sure that’s how to divide by 4?”
She looked at the problem, hesitated for a moment and said. “Oh no. There’s an easy way to divide by 4. Halve it and halve it again.”
I couldn’t argue with that process. She proceeded to halve 56 by writing down 2.5 and 3. Then she wrote 2.8 in the answer box. I almost slapped my forehead in despair.
After a few more minutes of remembering how to halve, she did eventually get to the point where she found that half of 56 was 28 and then half of 28 was 14. She wrote that in the answer box.
Not satisfied, I asked her, “what if it had been 56 ÷ 6? How could you have done that?” She looked at me, blankly. I think that she was a little disappointed that even though she had arrived at the correct answer I hadn’t showered her in praise.
“Divide by 6? I don’t have an easy way for that.” OK. She didn’t actually say those words, but I’m sure she was thinking them.
So I showed her the number line in the photo below. I showed her how you could count up in 4s or in groups of 4 to arrive at the answer. I showed her how it would also work for dividing by 6 or dividing by 7.

I didn’t really get that ‘wow’ moment I was hoping for. I think she begrudgingly accepted that maybe the number line had some merits. Of course counting up in this way requires good recall of times table facts – facts that she struggled to remember.
It is interesting to me that the first stage on Bloom’s Taxonomy of remembering seems to have been pirated away for this particular student. Where she couldn’t initially remember to halve and her poor recall of times tables facts limited her approach to this question, she could, by contrast, remember quite well that there are some ‘easy ways‘ for doing things in maths. This in turn limited her understanding of the principles of division and stopped her applying any knowledge she had to this problem.
It seems to me then that we need to stop teaching tricks and easy ways that fill up children’s memories. We need to teach children to recall and remember important facts first, such as how to halve and double and times table facts. Then we need to teach children understanding, such as what division is – that it is both grouping and sharing (depending on the context). Then we can give them opportunities to apply their knowledge.
Love this post… This kind of analysis of a child misconceptions, and what actually happens when they are confronted with a problem that they cannot solve, is invaluable, I think, and something that does not happen enough in teaching. Your conversation with the girl in your class really reminds me of similar exchanges in John Holt’s “How Children Fail”; what is shocking to me, though, is that in the 50 or so years since that book has written, we are still in a position where children are pushed through school with a very limited understanding of basic mathematical concepts, with teachers instead focusing on “easy ways” to perform calculations (or, as Holt calls them, “recipes”), presumably because these are easier to teach than a secure conceptual understanding.
Echoing your metaphor of building on shaky foundations, one of my favourite passages from “How Children Fail” is still relevant, I think:
“Now Edward’s former teachers gave him many hours of special, individual ‘help’ on arithmetic. But their help consisted in trying to get him to learn the recipes for the problems that he was supposed to know how to do. None of them tried to find out, as for years I never did, just what he did know about numbers, what sort of mental model he had of the world of numbers and how they behaved. As a matter of fact, this boy, if he is feeling good, can carry out correctly quite a number of arithmetic recipes; he is by no means the worst in the class in this respect. But this knowledge is apparent, not real.
The distinction is vital, yet many teachers do not seem to know that it exists. They think, if a child doesn’t know how to multiply, you show him how, and give him practice and drill. If he still makes mistakes, you show him again, and give more practice. If, after you have done this about a dozen times, he still makes mistakes, you assume that he is either unable or unwilling to learn – as one teacher put it, either stupid, lazy, disorganized, or emotionally disturbed. We do not consider that such a child may be unable to learn because he does not grasp the fundamental nature of the symbols he is working with. If numbers themselves are meaningless, how can multiplication be meaningful? Trying to teach such children to multiply, divide, etc., is like trying to build a ten-storey building on a foundation of old cardboard boxes. With the best will in the world, it can’t be done. The foundation must be rebuilt first. Children like Edward, and there are many, would not be in the spot he is in if, all along the line, their teachers had been concerned to build slowly and solidly, instead of trying to make it look as if the children knew all the material that was supposed to be covered.”
Thanks for the comment James.
At a lecture on the MaST programme that I’ve recently been on, I was struck by the thought that poor maths teachers use the same technique as the English abroad. If they don’t understand it the first time they just say it again, louder and slower. Most adults with maths anxiety (including many primary teachers) can trace it back to a single incident of poor maths teaching between the ages of 9 and 11. One example quoted to me was of the teacher raising his voice and saying to the child “why don’t you just get it?” To me this just shows poor subject knowledge – we need to raise our subject knowledge as teachers so that we don’t find ourselves being unable to teach difficult concepts.
I like the English abroad analogy… And I agree with you about subject knowledge, but I think in primary teaching this idea is often misinterpreted – very often, when teachers are anxious about their own ability in maths, they talk about it as though this is only an issue at the top of Key Stage 2 (I’ve heard lots of teachers say things like “I wouldn’t be very confident teaching Year 6, as I don’t think my maths is good enough”, implying that their maths is therefore “good enough” for Year 4, or wherever). For me, this misses the point that “subject knowledge” entails not only being able to do something yourself, but having a secure enough understanding of how and why it works, and the progression of skills involved in learning how to do it, to be able to teach it to others.
In my view, whether the maths involved is Level 2 or Level 5 is irrelevant – just because all teachers would be confident in their own ability to, for example, order 2-digit numbers, or carry out simple division, doesn’t mean that they are all able to teach these concepts in such a way that children will fully understand them before they are moved on. In fact, I’d argue that this is even more of a problem in Key Stage 1, where (if misconceptions are glossed over, and children rushed onto more abstract operations, like the “tricks” or “easy ways” you describe, before they are ready) the shaky foundations that you talk about are often first built.
To quote Holt again, “There is nothing particularly simple about ‘simple’ arithmetic”. I think the key at primary school level is acknowledging the complexity of what might appear to us, as adults, to be basic concepts, and trying to see these concepts through the eyes of the children, being aware of the (often very superficial) prior knowledge and understanding that they bring to them.